How can Corner Radius be Used to Increase Speed?

Did you know that you can calculate the radius of a corner just by having speed and lateral g’s? The formula is pretty simple. Here is the one that I use in AIM software:

((“GPS Speed”*1.46667)^2)/(“GPS LatAcc”*32.17405)

Speed is in mph and lateral acceleration is in g’s. Multiplying the speed (mph) by 1.46667 gets the speed in ft/s and multiplying the lateral g’s by 32.17405 gets the g forces in ft/s². The radius of the corner then ends up being in ft. It is amazing how accurate this formula can be. In Exhibit 1 I used Google Earth to look at turn one at VIR and I drew a circle that has a radius of 122 ft.

Exhibit 1

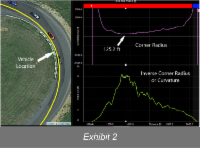
Exhibit 2

But the corner radius value doesn’t mean as much to me as the shape of the trace because the shape is effectively telling you when the car has turned and how sharp it has turned – or when the car has stopped turning. Please excuse my poor attempt and drawing the three different shapes that indicate what type of apex the car has taken but Exhibit 3 shows the general corner radius shapes indicating

early, mid, and late apexes as would be seen on a time-distance graph. These traces are assuming the track width at corner entry and corner exit are the same.

Exhibit 3

The reason for the shapes is because if a driver turns in early then they are going to have the most steering input exiting the corner and if they apex late then they are going to have the most steering input entering the corner. Exhibit 4 shows three more attempts at drawing and hopefully these make it a little easier to understand.

Exhibit 4

It is hard for me to find “clean” examples of what these corner radius traces in the data but Exhibit 5 shows some that I found.

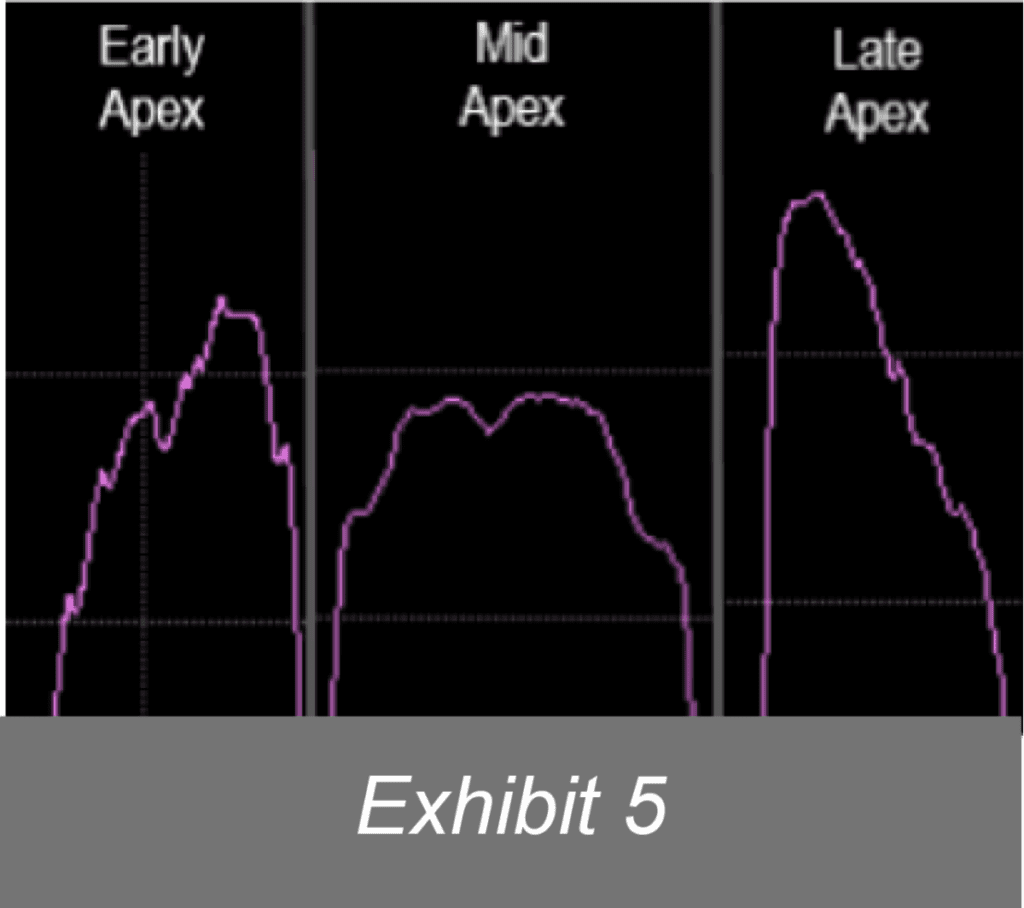
Exhibit 5

Since these traces are showing when and how much the car is turning, it is also a good way to see if a driver ran out of room by apexing too early, missing the apex, or just using an extra bit of pavement that might be available for a limited distance. This will show as a “bump” towards the end of the trace because the driver will have to turn the car again.

The Benefits of Corner Radius Calculation

But there is another advantage to having the corner radius calculation, and that is because by taking the inverse (dividing the corner radius value into 1) then it will tell you the exact point where the car rotated the most. This value is also known as the Curvature. This is shown in the green trace in Exhibit 2.

If you remove the Google Earth background in AIM RS3 Analysis, then you can choose a color channel for the driven line. In Exhibit 6, I used the Inverse Corner Radius or Curvature as the color channel. This shows that the tightest radius that the car was on was 119 ft and the exact point of the track where that happened. If there was another lap where the driver was faster through this corner and the driver apexed the corner at a different point, then by using the Inverse Corner Radius trace it would be easy to measure the distance between the two apexes and understand better why one apex point is better than the other.

In addition, please note that the steering trace has been added to Exhibit 6 as well. You can see that there is definitely some correlation between the Inverse Corner Radius and the Steering Angle but it is also clear to see that the car rotated more at corner entry since the slope of the Inverse Corner Radius trace is steeper, but the driver did not have to turn the steering wheel much to get the car to rotate. This indicates that the car had some oversteer as can also be seen with the steering corrections in the middle of the corner.

Exhibit 6

One item of note if you try to recreate this math channel, the values while the car is going straight are VERY large and the easiest way to deal with that is to change your scaling so that it is between -1000 and 1000 since the corner radiuses that we are concerned about are going to be well less than a 1000 ft. You can even use -500 to 500 for the scale if you like.

Learn More about Increasing Speed with Corner Radius

We are Precision Driving Analytics and we hope this helped you further understand how go faster using corner radius calculations. So the next time you are curious about how tight a corner is or if you want to figure out which apex point results in the best time through a corner, look no further than the Corner Radius calculation!

Call (948) 257-0556 or click here to contact me today.